Stevin - Arithmetic
D I S M E
Introduction: 'Principal Works' (PW) II A, 373-385. Some remarks: The first web-edition of De Thiende, with beginnings of La Disme, of Disme, and of Chr. Dybvad, Decarithmia (Danish, 1602) was: 'Honkblad van Simon Stevin' (1997).
Many, seeing the smalnes of this Book, and considering your worthynes, to whom it is dedicated, may perchance esteeme this our conceyte absurd: But if the proportion be considered, the small quantity hereof compared to humane imbecility, and the great utility unto high and ingenious intendiments, it will be found to have made comparison of the extreame tearmes ...The smallness of the book*) does not seem to be in accordance with the worthyness of the gentlemen. But consider the proportion: |
small quantity : human weakness = utility : understanding
1 2 3 4
|
|
One should not compare the extremes 1 and 4, but the third and the fourth. *) Stevin, 1585: 16 cm, 36 p., but Norton, 1608: 28 cm, 42 p.
some may say that certaine inventions at the first seeme good, which when they come to be practized, effect nothing of worth, as it often hapneth to the serchers of strong moving, which seeme good in small proofes and modells, when in great, or comming to the effect, they are not worth a ButtonStevin had 'roersouckers' in Dutch, and 'chercheurs de forts mouvemens' in his own French translation — probably used by Norton. Is it about instruments of force? Stevin described one in his Weegdaet (1586): the all-powerful 'Almachtich' [p. 37], referring to the 'Charistion' of Archimedes [>]; and in 1658 Gaspar Schott explained the 'Pancratium infinitae potentiae Simonis Stevini' in his Magia universalis naturae et artis [>]. Or maybe it is about the 'perpetuum mobile' or "eeuwich roersel ... t'welck valsch is", which is false (Weeghconst, p. 42).
NotationThe cumbersome notation of Stevin, with after each decimal a numeral in a circle (here: between brackets) has been explained by George Sarton (Isis, 1935, 175) as follows. In the explication of the third definition (p. C2):8 (0) 9 (1) 3 (2) 7 (3) are worth 8 9/10 , 3/100 , 7/1000Sarton: "Let us write this more explicitly 8 (1/10)0 9 (1/10)1 3 (1/10)2 7 (1/10)3then let us cancel the repetitious (1/10), and let us agree to represent (1/10)n by (n). This shows that Stevin's decimal notations were really decimal exponents, and explains if it does not justify, the ambiguity which he permitted to exist between his decimal and his algebraical symbolisms." For in L'Arithmetique something as 3 (2) + 9 (1) + 5 (0) means: 3 x2 + 9 x + 5. This explanation seems plausible, although it may be said that a zero power as (1/10)0 is not found in Stevin's work. Cf. Albert Girard, l'Algebre (1629) p. [16], 'Des Caracteres des puissances & racines': 18 (0) is the same as (1) 18, the first being 18 times 1, en the second 181. Sarton, p. 184: a quotation showing that different systems of notation were used at the end of the 17th century. And p. 186: "As compared with the whole series of later textbooks the Thiende stands out with all the austere beauty and dignity of an Archimedian monument."  Stevin's notation was used by Jacques Ozanam, in a 'Traité de la dixme, ou des fractions decimales', in L'usage du compas de proportion, 1691, p. 212. Source: 'The Erwin Tomash library' (2009), cat O, 70. Bernard Lamy, Elemens des mathematiques (1692), p. 284: "entiers .. 2' vaut deux primes, 5'' vaut cinq secondes, 1''' une tierce ...". A 'body of one yard' (p. D4v) is a cubic yard, and includes 1000 cubes with a side of 1 (1) or 0,1 yard. So 0,1 cubic yard includes 100 cubes with a side of 0,1 yard. To surveyors a 'rood of land' was a square rood of land, but with the 'foot' as a measure of surface it was different, that could mean a strip of 1 rood by 1 foot: when they say 2 Roodes, 3 Feete of Land, it is not barely meant 2 square Roods, and three square feete, but two Roods (and counting but 12 feete to the Rood) 36 feete squareIn the Meetdaet (Practice of measurement) Stevin says that surveyors make a difference between 'riem-voeten' (strip feet) and square feet [>]. DecimalFor Measurers decimal numbers are not to be set aside, as Stevin later made clear in his Meetdaet (1605), written for Maurice of Orange. In the preface he says [>] about the decimal notation [my translation]:... his Princely Grace (as being more than ordinarily experienced in it) ... has said several times that he finds in it such suitability and certainty, that the operations with it done easily by him, would not be performed otherwise by broken numbers without spending troublesome labour, more than would be useful.In 1600 Johan Sems en Jan Pietersz. Dou (Practijck des Lantmetens, p. 2) say that as a measure of length they will use "principally the rods, and the tenth parts of the rod (as being the most easy and practical to calculate with)." On p. 5: "a Surveyor's foot, or a tenth part of a rod". But they do not use Stevin's notation of decimal fractions (example: p. 33). Adriaan Metius, Manuale arithmeticae et geometriae practicae (1633), p. 13: "Om het overschot van divisie in tienden te brengen, in de Geometria gedienstich." (To bring the rest of a division to tenths, useful in geometry.) As an example the fraction 312/2864 is made decimal: put three zeroes behind the numerator and divide, then write 1' 0" 9"', and say 1 scr. prima, 0 scr. secunda, 9 scr. tertia (scr. - scrupula). On p. 19: "Soo ist mede dat in de practijck der Geometriae de oude Mathematici hare mate ... ghedeelt hebben in tienden, waer door sy haer roede oft mate hebben genaemt Decempedam". (Also the ancient mathematicians in the practice of geometry have divided their measure in tenths, so they called their rod or measure 'Decempeda'.) The Roman 'pertica' was 10 feet, see for example Balbus [6]: "Decempeda, quae eadem pertica appellatur, habet pedes X". A surveyor was sometimes called a 'decempedator' (^). Johan Stampioen, Algebra ofte nieuwe stel-regel (1639), p. 5, takes for the signs of powers "those signs with which the tenth arithmetic is usually accomplished, as well in Surveying as in Fortress building", that is (1), (2), etc., as Stevin did in L'Arithmetique. Then 7 (1) + 12 means 7 x + 12, and 1 (1) stands for x. It is true that sometimes Stampioen uses those signs for numbering digits (example: p. 51); but fractions he notates in the old manner, even when the denominator is a power of 10. André Tacquet, Arithmeticae theoria et praxis (1656 .. '83), p. 171: "quemadmodum dividendi labor per Neperi laminas rabdologicas, & logarithmos prope omnis evanuit; ita molestiis fractorum Simonis Stevinii praeclaro invento liberati sumus". Tacquet's notation is: I, II etc. above tenths, hundredths etc. Frans van Schooten, Mathematische oeffeningen (1659), I, puts (1) behind the first decimal, etc. (p. 27, 41, 54), but has also broken numbers (p. 99-102: alternatively). He writes 'aaaa' as a4 (V, 350, 434-), the notation of Descartes in La Geometrie (Discours ..., 299). Wine rodAbout wine gauging (p. D3-) there is a Wikipedia-article in Dutch: Wijnroeier.Stevin shows his skill in explaining with the subdivisions of the wine rod, "into 10 equall parts (namely, equall in respect of the wine, not of the Rod; of which the parts of the profunditie shalbe unequall)". Norton's figure on p. D3v is less clear than Stevin's on p. 28. No reason is given why one should take the 'mean proportional', but: We have made the demonstration briefe, because wee write not this to learners, but unto masters in their science.Others who studied wine gauging (without using decimals): - Nicolaas Petri, Practicque om te leeren reeckenen ... (1583/1591), fol. 212v-218 (256v-267, with tables). - Adriaan Metius, Arithmeticae et Geometriae practica (1611): a drawing of the measuring of a wine barrel (see 1626, Geom. p. 198 for his explanation of the wine rod or 'visier-roed' and a table of numbers; and: Manuale, 1633, p. 139-). - Michel Coignet, La géometrie (1626): a drawing for approximation as a cylinder (see p. 56 in the book). - Isaac Beeckman, Journal, III, p. 77-79 (1628): a simplified little table "hetwelcke men gemackelick van buyten leeren kan of op synen stock teeckenen" (which is easy to memorize or draw upon one's stick). In the beginning of the 16th century the number zero was still a problem, but around 1600 wine gaugers started to use decimal numbers (not merchants: in 1604 Willem Bartjens did not mention them). Visierbüchlein (Bamberg 1485). Heinrich Schreyber (Grammateus), Eynn kurtz newe Rechenn unnd Visyr buechleynn (1523) needed a whole page for explication of the zero with whole numbers: "Steht sy vor acht so wirt achtzigt als 80. acht zehen mal gesatzt." (If it stands before eight, then eighty is put as 80, eight ten times). Idem, Conometria Mauritiana (1619); Das ist, Ein newer Stereometrischer Tractat, von der lang-gesuchten unnd gewündschten Visierung deß vollen unnd lähren Stücks, oder Theyls eines Weinfasses. Op p. 64b: 'Abriß der Circulruthen' (zie p. 66). On p. 12 a reference to his Logistica decimalis: Das ist: KunstRechnung der Zehentheyligen Brüchen, (1619). The name of Stevin is missing there in the index of persons. Idem, Kurtzer Bericht von Zubereytung einer Visier-Ruthen auss einem geeichten Weinfass (1620). Joh. Kepler, Nova stereometria doliorum vinariorum (1615). Opera omnia: IV, 551-646. Idem, Ausszug auss der uralten Messe-kunst Archimedis, or 'Oesterreichisches Wein-visier-büchlein' (1616), in Opera V, 497-613; 'Zehnerzahl' and Jost Bürgi on p. 547. (^) (^)  Melchior Oechsner, Visierkunst (1616), Nemlich Wie man auß rechtem gewissen Grunde, auff eine jegliche Ohm, unterschiedliche VisierRuthen machen und dardurch eines jeglichen cörperlichen Dinges inhalt erfinden sol.
Melchior Oechsner, Visierkunst (1616), Nemlich Wie man auß rechtem gewissen Grunde, auff eine jegliche Ohm, unterschiedliche VisierRuthen machen und dardurch eines jeglichen cörperlichen Dinges inhalt erfinden sol. (1 'Ohm' or aam/ame is ±150 l. Stevin is not mentioned.) There is an 'Eyghentlicher Abriß der Viesier Ruthen'. On p. 5: "... alle Zahlen in gleicher Ordnung von 10. zu 10. gradiren ...", after which follow operations with decimal numbers.  Christiaen Martini Anhaltin, Oprecht, grondich en rechtsinnigh school-boeck van de wyn-royeryen (1663) (^) uses decimal numbers from the beginning, like these square roots on p. 9:
Christiaen Martini Anhaltin, Oprecht, grondich en rechtsinnigh school-boeck van de wyn-royeryen (1663) (^) uses decimal numbers from the beginning, like these square roots on p. 9: In the second part he is fighting against Cornelis van Leeuwen, using his notation, which has a remnant of Stevin's, as on p. 45: 6184. 38700 (5). Tim Nicolaije, 'Dwaasheid of retoriek? Cornelis van Leeuwen en de "Belachelijke Geometristen"', in Studium 5-1 (2012) 1-14. A.J. Daub, Meten met maten (Walburg Pers, 1979), p. 72-3: explains the use of the wine rod. Ad Meskens, 'Wine gauging in late 16th- and early 17th-century Antwerp', Historia mathematica 21 (1994), 121-147. Daniel Burckhardt, 'Zur Fassrechnung Johannes Keplers' (2000). Zero: beginning of number, cipherThe zero was not yet an ordinary number, as is evident in the definitions [>] of Norton's introduction (taken from Stevin's L'Arithmetique): "The Characters by which Numbers are denoted, are ten; namely, 0 signifying the beginning of Number, and 1, and 2, ...", and "Number is that which expresseth the quantitie of each thing". Where there is no quantity, there is no number.Later, in the Wisconstige Gedachtenissen, Stevin gives zero another name — 'numerical point' in stead of 'beginning of number' — in historical contemplations about the 'Age of the Sages' [PW III, 601]: the Noble and Very Learned Mr. Josephus Scaliger has shown me that the Arabs drew a point for it, as follows: . , also calling it point, and these points were marked underneath*) the numerals, where we put 0But the name 'numerical point' (talpunt) has not been found elsewhere. It is somewhat confusing that 'commencement' (begin) was a name for 'whole number' in def. 2 of Disme: "Every number propounded, is called Comencement, wose signe is thus (0)." Cf. Sarton [<]: it is a decimal exponent.  John Napier, Rabdologiae, seu Numerationis per virgulas libri duo (1617); reckoning with rods (G: rhabdos - rod, L: virgula - small rod); on p. 21-22 Stevin is mentioned. According to Sarton (1935, p. 181) Napier was "the main introducer of decimal fractions into common practice".
John Napier, Rabdologiae, seu Numerationis per virgulas libri duo (1617); reckoning with rods (G: rhabdos - rod, L: virgula - small rod); on p. 21-22 Stevin is mentioned. According to Sarton (1935, p. 181) Napier was "the main introducer of decimal fractions into common practice". In the translation by Adriaan Vlacq, Eerste deel vande nieuwe Telkonst, 1626 txt the rods are called 'roetjes'; h. IV has a 'Vermaningh voor de Thiende Telkunst' (admonition for the tenth arithmetic). The last subtitle of this work is Stevin's De Thiende. Idem, Mirifici logarithmorum canonis descriptio, 1614 ... 1619 with 'Constructio', where on p. 6: "whatever is written after the period is a fraction, the denominator of which is unity with as many cyphers after it as there are figures after the period" (Engl. 1889, p. 8). Henry Lyte, The art of tens, or Decimall arithmeticke (1619). In the beginning he gives the 'digits': 1, 2, ... 9; only later there is a name for the character 0: When the Multiplier beginneth on the right hand with one cipher, or with many; and endeth on the left hand with the digit 1, as these numbers following, 10, 100, 1000 ... 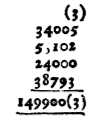 Lyte simplifies Stevin's notation of decimal numbers, by putting a numeral within brackets only at the end, as this addition shows.
Lyte simplifies Stevin's notation of decimal numbers, by putting a numeral within brackets only at the end, as this addition shows.
Note also that when you finde any ciphers on the right hand of any number that are not commencements*), you need not reckon of them, nor of their signes: as in the totall summe of this last example, which is 149900(3) which for brevities sake you shall say 1499(1) which is all one, as heere after shall often appeare.We now mostly write 149,9 for the result, but physicists are of the opinion that significant digits should be made explicit: 149,900. William Barton, Arithmeticke abreviated (1634), p. 1: "numbers consist of Nine figures and a cypher". On p. 19-23: images and explanation of 'Napeirs bones' (why is it that now-adays Napier's bones are not used in teaching arithmetic?); p. 115-7: 'To gage a barrell'. Noah Bridges, Lux mercatoria (1661), p. 1: "the little circle or cipher represented by the letter (0) signifies nothing, yet increaseth the value of a number, according to its place or position". Chap. 22 (p. 296-324): 'Of Artificial or Decimal Arithmetick'. "Although I am not of their opinion, who tell the world that all Arithmetical operations relating to Trade, are more speedily and easily performed by Decimal than Natural Arithmetick, yet am I no enemy to the Artificial part, where it is unforc'd and materially useful." On p. 302: 'The Tables in English Coin in Decimals'. On p. 308: "The decimals are not onely distinguished from the whole numbers by Commaes interposed between the parts, but for the Learners better observation pointed also." Weights and measuresNormalization does not happen of its own, and Stevin thinks that more has to be done than instruction alone (p. E2v):joyning the vulgar partitions that are now in weight, measures, and moneyes [...] that the same tenth progression might be lawfully ordained by the superiors, for every one that would use the sameHe was not the first with this opinion (PW, Intr. p. 383), nor the only one. Prince-elector Ernst of Bavaria had a plan for reforming the system of weights and measures, and in 1605 he asked the advice of Johannes Kepler about a manuscript, to which were added the opinions of Simon Stevin, Lazarus Schoner (^) and Adrianus Zelstius (^). In his response 'about equalization of measures' Kepler mentions Stevin. Opera omnia, V (1864), 616-626: '... de mensurarum aequatione ...', Pragae 24 Dec. anno 1605. [617] Tantum operae, tantum vigilantiae imponitur magistratibus omnibus a summo ad postremos, ut merito dubites, an operae pretium sit futurum haec lex perlata. Haec Stevinus tetigit, cum innuit, rem esse in imperio pene impossibilem, quo multae communitates merum imperium Caesaris de facto respuunt.Sarton does not mention it: Isis, 1935, 189, which is about Ernest and his plan. See also: Johannes Kepler, Gesammelte Werke, IX (1960), 540; Max Caspar, Kepler (1993), 159; Heinz-Dieter Haustein, Weltchronik des Messens (2001), 165. On Zelstius (Adriaan Zeelst) see: Koenraad Van Cleempoel, 'De Leuvense school van instrumentenmakers in de 16de eeuw', in R. Halleux, C. Opsomer, J. Vandersmissen, Geschiedenis van de wetenschappen in België (1998), p. 225-6, with the remark: "in de Biblioteca Medicea Laurenziana van Firenze wordt een manuscript bewaard waarin Zeelst samen met Lazarus Schoner meer uitleg geeft over gewichten, maten, proporties, munten en medailles, bestemd voor de Luikse prins-bisschop" (Ernst of Bavaria). So the first to propose the decimal mile based on the size of the earth ("ad maximum circulum globi Telluris") was Simon Stevin, not Gabriel Mouton. |

